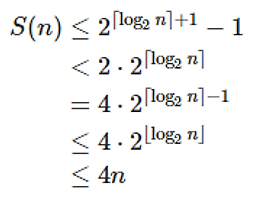11438번: LCA 2
첫째 줄에 노드의 개수 N이 주어지고, 다음 N-1개 줄에는 트리 상에서 연결된 두 정점이 주어진다. 그 다음 줄에는 가장 가까운 공통 조상을 알고싶은 쌍의 개수 M이 주어지고, 다음 M개 줄에는 정
www.acmicpc.net
## 최소 공통 조상
최소 공통 조상이란, 트리에 각 노드에서 공통된 조상을 갖을 때 가장 가까이 있는 노드를 의미한다. 만약에, 트리의 높이가 H라고 했을 때, 순차적으로 탐색을 한다면 O(H)만큼 걸릴 것이다. 문제의 조건에서 발생할 수 있는 H는 최대 N과 같으므로, 시간복잡도가 O(N * N)이 되므로 제한 시간안에 정답을 찾을 수 없다.
## 희소 행렬
위에서 높이를 O(H)에 순차적으로 접근하는 것이 아니라, O(logH)로 접근할 수 있는 방법이 있다. 내가 지금부터 설명하는 개념이 희소 행렬에 정확한 정의인지는 모르겠다. 구글링을 해봐도 희소 행렬에 대해서 정확하게 언급되어 있지 않아서, 문제를 풀면서 느낀 정의를 공유하고자 한다.
각 노드가 가지고 있는 부모 노드에 대한 정보는 순차적인 정보가 아니라, 2의 배수 승이다. 즉, 1번째 정보는 2^0이고, 2번째 정보는 2^1이고, 3번째 정보는 2^2승 ... N번째 정보는 2^(N - 1)이 된다.
각 노드들이 모두 동일하게 위의 정보를 갖고 있을 때, 모든 부모노드들을 방문할 수 있을까에 대한 의문이 당연히 있어야 한다.
우리가 알고 있는 사실, 모든 수는 이진수로 표현할 수 있다. 각 노드들이 가지고 있는 2^N의 정보는 이진 수의 각 수를 의미할 수 있다. 따라서, 노드들이 가진 2^N의 정보로 모든 노드들을 접근할 수 있다.
## 해설코드(Java)
|
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
|
import java.util.*;
import java.lang.*;
import java.io.*;
class Main
{
static BufferedReader br = new BufferedReader(new InputStreamReader(System.in));
static BufferedWriter bw = new BufferedWriter(new OutputStreamWriter(System.out));
static int N, M;
static ArrayList<Integer>[] alist;
static int[] depth;
static int[][] ac;
static int getPow(int num, int x){
int result = 1;
for(int i = 1; i <= x; i++)
result *= num;
return result;
}
static void getTree(int here, int parent){
int cur = here;
depth[cur] = depth[parent] + 1;
ac[here][0] = parent;
for(int i = 1; i <= 20; i++)
ac[here][i] = ac[ac[here][i - 1]][i - 1];
for(int i = 0; i < alist[here].size(); i++){
if(parent != alist[here].get(i))
getTree(alist[here].get(i), here);
}
}
public static void main (String[] args) throws java.lang.Exception
{
N = Integer.valueOf(br.readLine());
alist = new ArrayList[N + 1];
for(int i = 0; i < alist.length; i++)
alist[i] = new ArrayList<>();
depth = new int[N + 1];
ac = new int[N + 1][21];
for(int i = 0; i < N - 1; i++){
String[] sArr = br.readLine().split(" ");
int n1 = Integer.valueOf(sArr[0]);
int n2 = Integer.valueOf(sArr[1]);
alist[n1].add(n2);
alist[n2].add(n1);
}
depth[1] = 0;
getTree(1, 0);
M = Integer.valueOf(br.readLine());
for(int i = 0; i < M; i++){
String[] sArr = br.readLine().split(" ");
int n1 = Integer.valueOf(sArr[0]);
int n2 = Integer.valueOf(sArr[1]);
if(depth[n1] > depth[n2]){
int temp = n1;
n1 = n2;
n2 = temp;
}
for(int j = 20; j >= 0; j--){
if(depth[ac[n2][j]] >= depth[n1])
n2 = ac[n2][j];
}
int lca = n1;
if(n1 != n2){
for(int j = 20; j >= 0; j--){
if(ac[n2][j] != ac[n1][j]){
n2 = ac[n2][j];
n1 = ac[n1][j];
}else
lca = ac[n2][j];
}
}
bw.write(lca + "\n");
}
bw.flush();
}
}
|
'알고리즘 > 백준' 카테고리의 다른 글
| [BOJ 2357] 최솟값과 최댓값(Segment Tree, 세그먼트 트리, 자바) (0) | 2021.01.06 |
|---|---|
| [BOJ 1701] Cubeditor (백준, JAVA, KMP) (0) | 2021.01.02 |
| [BOJ 1655] 가운데를 말해요(Java, Time Complexity) (0) | 2020.12.23 |
| [BOJ 6591] 이항 숏다운 (0) | 2020.11.14 |
| [2019 카카오 개발자 겨울 인턴십] 징검다리 건너기(Java, 간단한 코드, Union-Find) (0) | 2020.09.07 |